
I heard that it matters what order you take your derivatives. Take a Tour and find out how a membership can take the struggle out of learning math. Sort by: Top Voted Questions Tips & Thanks Charles Leonard Lewis IV 10 years ago I was reading my calc book and they had (fg)' fg'+gf' which is the reverse of what Sal was saying. Still wondering if CalcWorkshop is right for you? Get access to all the courses and over 450 HD videos with your subscription
#CHAIN RULE CALCULUS WITH STEPS FULL#
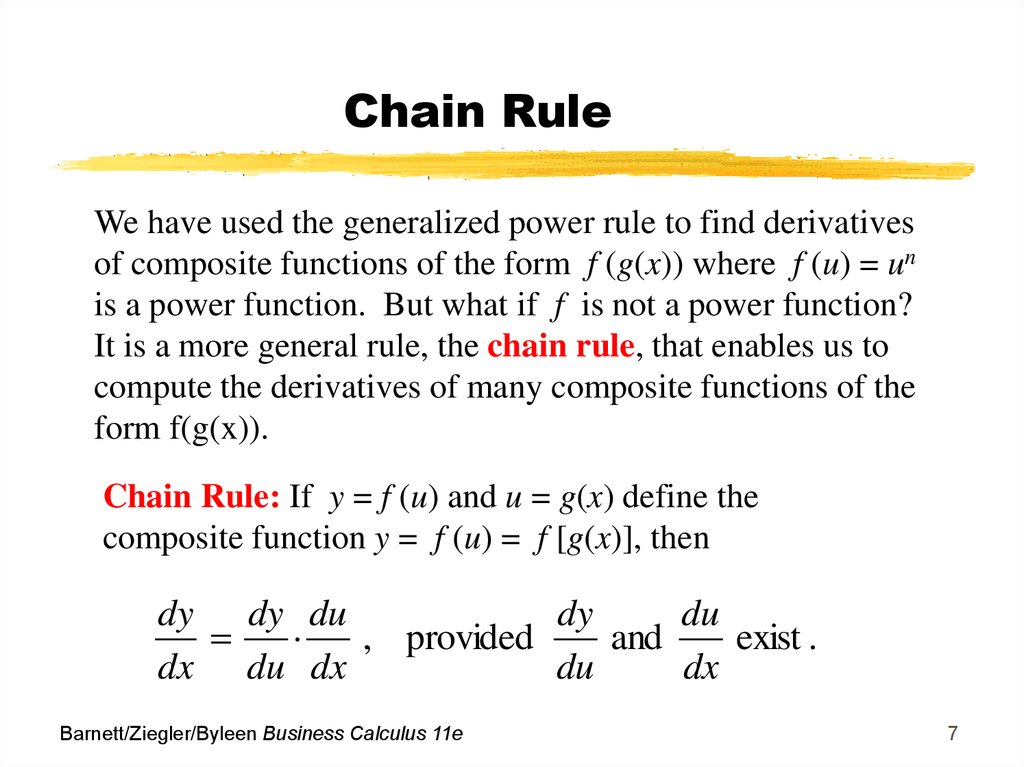
Let’s get to it! Video Tutorial w/ Full Lesson & Detailed Examples (Video) So, throughout this lesson, we will work through numerous examples of the chain rule, combining our previous differentiation rules such as the power rule, product rule, and quotient rule, so that you will become a chain-rule master! In fact, we will come to see that the chain rule’s helpfulness extends beyond polynomial functions but is pivotal in how we differentiate: Thanks to the chain rule, we can quickly and easily find the derivative of composite functions - and it’s actually considered one of the most useful differentiation rules in all of calculus. Step 3: Determine the derivative of the outer function, dropping the inner function. Step 2: Know the inner function and the outer function respectively. Good grief! That would have been painful. Step 1: Recognize the chain rule: The function needs to be a composite function, which implies one function is nested over the other one.
Then we multiply by the derivative of the inside function. Essentially, we have to melt away the candy shell to expose the chocolaty goodness. Without it, we would have had to multiply the polynomial you see in blue by itself 10 times, simplify, and then use the power rule to find the derivative! The Chain Rule formula shows us that we must first take the derivative of the outer function keeping the inside function untouched. Next, we multiplied by the derivative of the inside function, and lastly, we simplified. See, all we did was first take the derivative of the outside function (parentheses), keeping the inside as is. A special rule, the chain rule, exists for differentiating a function of another function.


 0 kommentar(er)
0 kommentar(er)
